Tetrahedron

Starting from triangle, quadrilateral and pentagon, there are infinite number of polygons. Polygons with all sides equal and all internal angles equal are called regular polygons and there are infinite numbers of regular polygons with 3 to infinite numbers of sides. In the limit the regular polygon becomes a circle.

Extending to the third dimension, there are infinite numbers of polyhedrons, starting from tetrahedron. Regular polyhedrons are those whose faces are all regular polygons congruent to each other, whose polyhedral angels are all equal and which has the same number of faces meet at each vertex. An interesting fact is that there are only five regular polyhedrons: the Tetrahedron (four faces of equilateral triangles), the Cube (six square faces), the Octahedron (eight equilateral triangular faces—think of two pyramids placed bottom to bottom), the Dodecahedron (12 regular pentagonal faces), and the Icosahedron (20 equilateral triangular faces). In this write-up, I want to concentrate on Tetrahedrons, (not necessarily regular ones), as an extension of triangle to three dimensions.

A tetrahedron is some times described as a triangular pyramid. It consists of four triangular faces and four trihedral angles. (Trihedral angles are discussed in good detail in my earlier blog). Even though three intersecting planes are enough to make a trihedral angle, they still do not make a closed polyhedron. A fourth non-parallel plan is needed to get a polyhedron of least order – 4 faces, 6 edges and 4 vertices; this is known as a Tetrahedron.

Let us consider the tetrahedron ABCD as above, for our further discussions, with BCD as the base triangle and A as the peak point. The areas of triangular faces may be denoted by Sa for triangle BCD opposite to vertex A,  by Sb for triangle CDA opposite to vertex B,  by Sc for triangle DAB opposite to vertex C and by Sd for triangle ABC opposite to vertex D. The dihedral angle between faces Sb and Sc and around the edge AD, may be represented by Φbc and respectively the other dihedral angles, Φac, Φad etc.

Volume of a tetrahedron

If we consider the tetrahedron as a triangular pyramid, with a triangular base of area S  and a height h, the volume of the tetrahedron can easily be derived as V = 1/3 S*h (just as area of the triangle is expressed as A = ½ (Base length)(Height)).

A Tetrahedron OABC is also described in terms of three non-co-planar vectors OA(a), OB(b) and OC(c). In this case the volume may be stated as V = 1/6^th (a.b x c). (i.e. One sixth of the volume of the parallelepiped a.bxc).

If Tetrahedron OABC is given in terms of lengths as (a, b, c) and angles between them as (α, β, γ) then, the above expression for volume can be expanded and simplified as:

V = (1/6) abc*√(1 + 2 cos α cos β cos γ  – cos²α – cos² β – cos² γ)

Two triangles ADB and ADC, with AD as the common edge, and Φbc as the dihedral angle between them, can fully describe the tetrahedron ABCD. Volume of this tetrahedron can be formulated as:

V = 2/3 (Area ADB * Area ADC) (Sin Φbc)/AD;

(or V= 2/3 Sc Sb Sin Φbc/AD)

Given opposite edges, AB and CD and the shortest (perpendicular) distance, EF(=d), between them, we can calculate the volume of the tetrahedron as below. Let Φ be the dihedral angle between the planes ABEF and CDEF. Then the volume of tetrahedron ABCD, V= (1/6) (AB) (CD) (EF) Sin Φ.

Law of Cosines and Sines

Law of cosines for a triangle ABC states: a² = b² + c² – 2bc cos A, where A is the angle between sides ‘b’ and ‘c’, opposite to side ‘a’.

Law of sines for a triangle ABC states: (Sin A)/a = (Sin B)/b = (Sin C)/c = 2S/abc, (where S is the area of the triangle).

Let us derive similar relationships for a Tetrahedron

In my earlier blog on Trihedral angles we have talked briefly about the Pythagoras relation for a right angled tetrahedron. We started off from a semi-right-angled tetrahedron as below: (AD is perpendicular to BCD). Mark an X such that DX and AX are perpendicular to BC.

Consider triangle BCD. We may write,

BC² = BD² + CD² – 2.BD.CD.cos Φ (law of cosines for triangle DBC)

Multiplying both sides by AD²

AD² * BC² = AD² * BD² + AD² * CD² – 2. (AD.BD)(AD.CD). cos Φ

(i.e.) (AX² – DX²) * BC²

= AD² *  BD² + AD² *  CD² – 2.(AD.BD)(AD.CD).cos Φ

(i.e.) (2Sd)² – (2Sa)² = (2Sc)² + (2Sb)² – 2(2Sc)(2Sb) cos Φ,

where Sj is the area of triangular face opposite to vertex j.

(i.e.) Sd² = Sa² + Sb² + Sc² – 2.Sb.Sc.cos Φ

This is the law of cosines for tetrahedron and in a more general form it is given as:

Sd² = Sa² + Sb² + Sc²

                  – 2.Sa.Sb.cos Φab – 2.Sb.Sc.cos Φbc – 2.Sc.Sa.cos Φca .

In addition to Φab = Φca = 90 degrees as above, if Φbc is also 90degrees (i.e.) for a fully right-angled tetrahedron (right solid-angled at D), we get the Pythogoras relation as,

Sd² = Sa² + Sb² + Sc²

For any trihedral angle Ω, G-sine Ω can be defined as, (refer to my earlier blog on Trihedral Angle)

G-sin Ω = Vp*Vp/ (Product of adjacent areas),

where, Vp is the volume of parallelepiped formed by the arms of the trihedral angle and areas are of adjacent parallelograms. Using this, for a tetrahedron ABCD we can write,

G-sine A = (Vp)²/(2Sb*2Sc*2Sd)

We know that, Volume of a tetrahedron, V, is 1/6^th the volume Vp of the extended parallelepiped. Hence,

G-sine A = (6V)²/(2Sb*2Sc*2Sd) = 9/2 (V)²/(Sb*Sc*Sd)

Similarly, G-sine B = 9/2(V)²/(Sc*Sd*Sa), and so on.

Hence we can write,

(G-Sine A)/Sa = (G-Sine B)/Sb = (G-Sine C)/Sc = 9/2 V²/(Sa.Sb.Sc.Sd)

Law of G-Sines for any tetrahedron can be simply written as

(G-Sine A)/Sa = (G-Sine B)/Sb = (G-Sine C)/Sc

      = (9/2) V²/(Sa.Sb.Sc.Sd), where V is the volume of the tetrahedron.

 

Centres of Tetrahedron

A triangle can be circumscribed by a unique circle known as circum-circle, which passes through all the three vertices of the triangle. The centre of this circle is known as circum-centre. Same way a triangle can inscribe a unique circle known as in-circle, which is tangential to all the three sides of the triangle. The centre of this circle is known as in-centre. In an equilateral triangle the circum-centre and the in-centre coincide to be the same point.

Just as a circle can be drawn through any three non-co-linear points in a plane, a sphere can be constructed to go through any four non co-planar points in space. Hence any tetrahedron can be circumscribed by a unique sphere known as circum-sphere, which passes through all the four vertices of the tetrahedron. Perpendiculars to each of the four triangular faces from their respective circum-centres are concurrent at the centre of circum-sphere, i.e. circum-centre of the tetrahedron.

However the tetrahedron may inscribe two kinds of spheres – one sphere which is tangential to all the four faces of the tetrahedron, and another sphere which is tangential to all its six edges. They are called in-sphere and mid-sphere respectively with their own in-centre and mid-centre.

In-Centre will be equidistant from all the four triangular faces. Consider a tetrahedron ABCD. Consider the dihedral angle Φcd around the edge AB. Consider a plane ABX bisecting this angle. Every point on this plane will be at equal distance from planes ABC and ABD. Similarly consider a plane ACX bisecting the dihedral angle Φbd around the edge AC. These two planes will intersect each other along a straight line AX through A. Any point on this line AX will be at equal distance from all the three planes forming the trihedral angle Ωa at A. One more plane, constructed to bisect the dihedral angle Φad around the edge BC, will intersect the line AX at a point X. This point X will be equidistant from all the four planes of the tetrahedron ABCD. And hence, X will be the in-centre of the In-Sphere which will be tangential to all the four faces of tetrahedron ABCD. Effectively, the in-centre of a tetrahedron is the point of coincidence of all the six planes, bisecting the six dihedral angles around the six edges of the tetrahedron.

Mid sphere itself can be visualized as a sphere jutting out of all the four faces but tightly caged by all the six edges of the tetrahedron. Mid centre will be equidistant from all the six edges. A bisector of a trihedral angle is defined as the locus of points that are equidistant from its (three) edges. Such bisectors of all the four trihedral angles of the tetrahedron will be concurrent at the mid centre. But do the mid-sphere and mid-centre exist for all tetrahedrons? This aspect is not discussed much in the existing literatures and hence I have discussed this in detail below. (This is a result of my own research)

Mid-sphere and Mid-centre of a tetrahedron

Perpendiculars to each of the four triangular faces from their respective in-centres, if they are concurrent, form the centre of the mid-sphere, i.e. mid-centre. Let us consider a tetrahedron ABCD, having a mid sphere tangential to all the six edges.  There will be three tangents to the mid-sphere from A. Let their lengths up to the points of tangency on the sphere be a1, all equal as they should be. Similarly on other three tangents each from B, C and D, let these equal lengths be b1, c1 and d1. We can easily observe,

AB = a1+b1; Similarly, CD = c1+d1; Hence,       AB + CD = a1+b1+c1+d1;

Similarly, AC = a1+c1; BD = b1+d1; and hence, AC + BD = a1+c1+b1+d1;

Similarly, AD = a1+d1; BC = b1+c1; and hence, AD + BC = a1+d1+b1+c1;

We see that, the Mid-sphere exists for a tetrahedron only if lengths of each of the three pairs of opposite edges add up to the same value.

Let us see whether the converse is true. Consider two triangles ADB and ADC, with AD as the common edge, and Φbc as the dihedral angle between them, which can fully describe the tetrahedron ABCD.

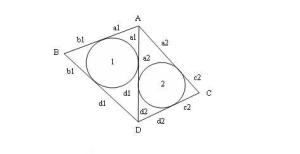
AB and CD form one pair of opposite edges of the tetrahedron. AC and BD will form the second pair and AD and BC (when folded across AD) will form the third pair. Let the lengths of each of these pairs of edges add up to the same value, k. (i.e.) AB+CD = AC+BD = AD+BC = k. Let the circles 1 and 2 shown above, be the in-circles of the respective triangles. Let a1 and d1 be the lengths of tangents from A and D to circle-1. Similarly, let a2 and d2 be the lengths of tangents from A and D to circle-2. Same way, the tangents from B and C are also shown above as b1 and c2.

Now, AB + CD = a1 +b1 +c2 +d2 = k.

Same way, AC + BD = a2 +c2 +b1 +d1 = k.

Hence (a1 +d2) = (a2 +d1). This is possible only when circles 1 and 2 touch each other at a common point on AD. This common point and the in-centres of 1 and 2 will all lie in a plane perpendicular to AD, This means, the perpendiculars from the in-centres of 1 and 2 to the respective faces will meet at a point, say Om. Similarly considering other pairs of opposite edges, we may conclude that each adjacent pair of the four in-circles on the four faces of the tetrahedron will be tangential to the respective common edge at the same point. And hence the perpendiculars to the respective faces from their in-centres will all be concurrent at the mid-centre, Om, which will be equidistant from all the edges. Hence we have a mid-sphere also.

Hence a tetrahedron ABCD will have a mid-sphere if and only if the lengths of each pair of opposite edges add up to the same value. Any tetrahedron with equilateral triangle as the base and with other three edges of equal lengths, easily satisfy this requirement and they do have mid-spheres and mid centres. There can be many others also satisfying this condition and having a mid sphere. For a regular tetrahedron, all the three centres, i.e. , Circum-centre, Mid-centre and In-centre will coincide.

The centre of gravity (Centroid) of a tetrahedron is well defined. A median of a tetrahedron is defined as a line from any vertex to the centroid of the opposite triangle. All the four medians of a tetrahedron are concurrent at the centroid of the tetrahedron. Consider the three straight lines connecting the respective mid points of opposite edges of the tetrahedron. It is interesting to note that the centroid is also the point of concurrence of all these three bi-medians, as they are called. The centroid divides all the medians in the ratio of 3:1 (just as ratio of 2:1 for triangles) and divides the bi-medians equally.

However ortho-centre is not defined for a tetrahedron as the four orthogonal lines need not always be concurrent.

Conclusion:

Tetrahedron has really turned out to be an interesting solid. There are many more interesting features which may hopefully come out as comments from my readers. Perhaps my next blog could be on parallelepiped (or hexahedrons, in general).

Additional Reference:

http://mathworld.wolfram.com/Tetrahedron.html

 

Addendum

There was a request from one of my readers, Shivangi verma

I want a solution for this question
1.Show that the three lines joining the mid points of edges of a tetrahedron meet in a point which bisects them?

Plz provide me with the solution asap…

Here is my proof.

Centre of Gravity CG of a tetrahedron

In the following figures, tetrahedrons are shown like a open triangular book. The first one is shown open along the edge CD. In the second one same tetrahedron is shown open alone the opposite edge AB.

N and M are the midpoints AB and CD, the opposite edges of the tetrahedron. G1 is the cg of ∆ACD and G2 is the cg of ∆BCD, the two adjacent faces of the tetrahedron. |||’ly, in the other figure, G3 and G4 are cgs of ∆s CAB and DAB, the other two adjacent faces of the tetrahedron. The medians of tetrahedron, AG2 and BG1 intersect at Ga, on plane AMB. This is known as cg of the tetrahedron. |||’ly, Gc the cg of tetrahedron as seen from C and D, will be on the plane CND. We are required to prove:

1. Ga and Gc coincide
2. They do so, at the midpoint of ‘Bi-median’ MN.
3. We are also required to prove all the three ‘Bi-medians’ are concurrent at their midpoints which is also the cg of the tetrahedron.
4. In the process we will also prove that CG of tetrahedron divides each median in the ratio 1:3.

Please refer to the following  of ∆ABM, extracted from the above diagram of the tetrahedron.

Now, Area ∆AMN’/Area ∆BMN’ = Area ∆AGaN’/Area ∆BGaN’ = AN’/BN’

Then it follows, Area ∆AMGa / Area ∆BMGa = AN’/BN’   ——- 1

|||’ly,   Area ∆AMGa / Area ∆ABGa = MG2/G2B = 1/2   ——- 2

And     Area ∆BMGa / Area ∆BAGa = MG1/G1A  = 1/2   ——- 3

G1 and G2 being the centroids of triangular faces, we know MG2/G2B = MG1/G1A = ½,  as written above, and G1G2 is ||lel to AB.

Dividing Eqn-2 by Eqn-3,  We get, Area ∆AMGa / Area ∆BMGa = 1

i.e. AN’ = BN’. (i.e.) N’ coincides with the midpoint N.

Hence Ga lies on Bi-Median MN.

|||’ly, by extracting ∆NCD  from the second diagram of tetrahedron, We can prove Gc, the CG of tetrahedron as seen from C and D will also be on the Bi-Median MN.

Hence We have proved that (a) all four medians of tetrahedron from its vertices A, B, C and D are concurrent on the Bi-Median MN.

Again from Eqns 2 and 3, we know, Area ∆AMGa = Area ∆BMGa = ½ (Area ∆ABGa)

Therefore, Area ∆ABGa = ½ (Area ∆ABM)

Hence we may conclude, Ga is the midpoint of Bi-Median MN

|||’ly, from ∆NCD, We can conclude, Gc also is the midpoint of Bi-Median MN

Hence Ga and Gc coincides with G0, the midpoint of MN, which is the CG of Tetrahedron.

Hence we have proved the CG of tetrahedron coincides with midpoint of the Bi-Median MN.

Similarly if we consider AC and BD, the CG of tetrahedron (obtained from the pairs of medians from A,C and B,D) will again coincide with the midpoint of the line joining the mid-points of edges AC and BD).

Similarly if we consider AD and BC, the cg of tetrahedron (obtained from the pairs of medians from A,D and B,C) will again coincide with the midpoint of the line joining the mid-points of edges AD and BC).

This clearly proves both the facts:

1. All the 4 medians from the four vertices of tetrahedron are concurrent at one point known as CG of tetrahedron, which also is the concurrent midpoint of all bi-medians.
2. All the three bi-medians from the three pairs of opposite edges are concurrent at their midpoints which also is the CG of tetrahedron

Now, draw A’B’ parallel to AB through Ga. As Ga is the midpoint of NM, A’ and B’ also become midpoints of AM and BM respectively.

i.e., MA’/A’A = 1. But we know, MG1/G1A = ½

For simplicity, let us assume, MA= 6k.

Then MG1 = 2k and G1A = 4k as G1 is a centroid. And MA’ = 3k as A’ is the midpoint.

Hence, G1A’ = 1k and A’A =  3k.

i.e.,  A’ divides  G1A in the ration 1:3. |||’ly, B’ divides  G2B in the ration 1:3.

Hence we may conclude, Ga also divides the medians BG1 and AG2 in the ration 1:3. Same will be true of Gc, with other medians DG3 and CG4.

Phew! That was interesting!

– L V Nagarajan 27 June 2017

 

 

 

Tags: Geometry, Maths